Distance and displacement
Distance is a concept familiar to you. When you go from home to school, you have to travel a certain distance. Sometimes, there can be several paths you could use to travel between home and school. Some of them could be shorter and some longer. Figure 2.1 shows several paths that a child could take, to travel from a point A to another point B.
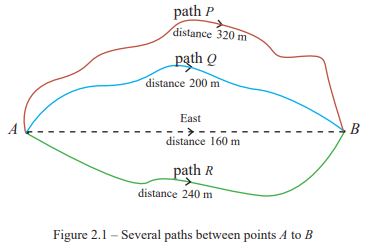
If path P is used, the distance between point A to point B would be 320 m. If path Q is used, this distance would be 200 m. If path R is taken, the travel distance would be 240 m. This shows that the distance depends not only on the starting and the end points, but also on the path used to traverse the distance.
Whichever the path the child uses to reach B after starting from A, the ultimate result is that he has moved a distance of 160 m from A to B on a straight line towards the east . A change of position like this, that occurs from one point to another point in a particular direction is called displacement. The magnitude of the displacement is the shortest distance between the two points.
In the example above, the displacement of the child is 160 m to the east. Although the distance has changed according to the path taken, the displacement has remained the same.
In addition, there is another important difference between distance and displacement. Because we do not take the direction into account when measuring the distance, distance has only a magnitude. It does not have a direction. Therefore, distance is a scalar quantity. However, when measuring the displacement, the direction is important. In other words, the displacement has both a magnitude and a direction. Therefore it is a vector quantity.
Let us understand this concept further with following examples.



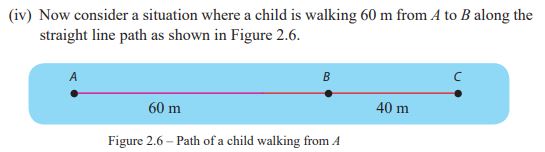
The displacement of the child is 60 m along AB. Thereafter, if the child walks another 40 m along the same direction and reaches point C, what would be his total displacement?
When two or more displacements occur along the same direction, they can be either added or subtracted using ordinary arithmetic.
Since the displacements in the above example are in the same direction, total
displacement = 60 m + 40 m = 100 m
This means that the child is now at a point 100 m away on a straight line from the starting point.
Now suppose that the child walks back 40 m after reaching the point B, as shown in Figure 2.7, instead of walking forward. The displacement corresponding to 40 m is in the opposite direction to that of AB. According to the figure 2.7 it is clear that the total displacement is given by the line AC. Therefore, although the distance traversed in this case too is 100 m, his displacement would be 60 m + (-40 m). That is 20 m.

If he walked the same distance in the opposite direction after reaching the point B, his displacement would be 60 m + (-60 m). That is, a zero (0) displacement. From this we know that the child is back at the starting point.